Everything in the universe that has mass, has gravity. It’s easy to understand that the Earth, large as it is, has gravity, which pulls on us constantly, keeping us on terra firma. It’s just as easy to understand that other large objects have gravity, like the Moon, planets, and the Sun.
However, it’s much harder to understand that every person on Earth has gravity. Strangely, you exert a force on the Earth, and on every other human. You also exert a force on every rock, tree, and creature that roams the Earth, and they all exert a force on you. The only problem is that you have such a small mass compared to the Earth, the gravitational force you exert is very tiny.
When I explain this idea, I’m often asked “Why don’t we feel the gravity from a mountain?”
For one, it’s because the Earth itself weighs much more than the mountain, so the mountain has little gravity, even though its huge. The other reason is that the gravity exerted by any spherically distributed mass (like the Earth) behaves as if all the mass was at the center of the object. If you’d like to see the actual math, check this link for the breakdown.

If a mountain can’t exert enough force for us to feel, what about the Moon? The Moon is big, and it’s relatively close to us. What if the Moon is overhead? Would it’s pull make us lighter? Would it make us heavier if it was on the far side of the Earth?
Let’s figure it out!
Isaac Newton first figured out the force of gravity and how it works. He came up with an equation to measure the gravitational force between any two objects:

Where r is the distance between the objects’ center of mass, and G is the universal gravitational constant, where G = 6.67 x 10-11N(m/kg)2.
You, standing on the Earth!
The Earth weighs 5.72×1024 Kg, and the distance between you and the center of the Earth (where you feel the pull of gravity) is r = 6,371,000 m. Throw all this into the equation above and we find that the force exerted on you from the Earth is:
F = 9.4 x Myou
So ~9.4 times your mass. This is why more massive things are harder to lift. The actual answer, measured by high quality experiments, is 9.81 times your mass. It’s different than our calculation due to the fact that the Earth doesn’t have the exact same density everywhere (some of it is water, rock, etc). If you divide this force by your mass, you get the Earth’s acceleration due to gravity, independent of mass:
g = 9.81 m/s2
All things accelerate at the same rate, no matter how heavy they are. A feather falls as fast as a bowling ball. The only difference is that the bowling ball will hurt a lot more if it falls on you.
Now the Moon
The Earth and Moon are orbiting a point between them, their center of mass. Since the Earth is more massive, the center of mass of the Earth-Moon system is inside the Earth, about 1,700 Km below the surface. This means the Earth ‘wobbles’ as the Moon orbits.

The Earth and Moon are actually in free fall around that Center of Mass. It’s the same as astronauts in a spaceship orbiting the Earth. They are falling around the Earth in an ellipse, but without a stationary surface to push on them, they feel weightless. Their net force is the centripetal force, which is equal to the gravitational force. Centripetal force is given by:
F = m*v2 / r
You and the Earth are both in free fall around the center of mass. But because you are stationary on the Earth, the ground pushes on you and you perceive the Earth’s gravity.
“But I thought ocean tides happen because of the Moon’s Gravity!”
They do! When we talk about the interaction of two large bodies, such as the Moon’s gravitational influence on the Earth, we need to discuss the tidal force.
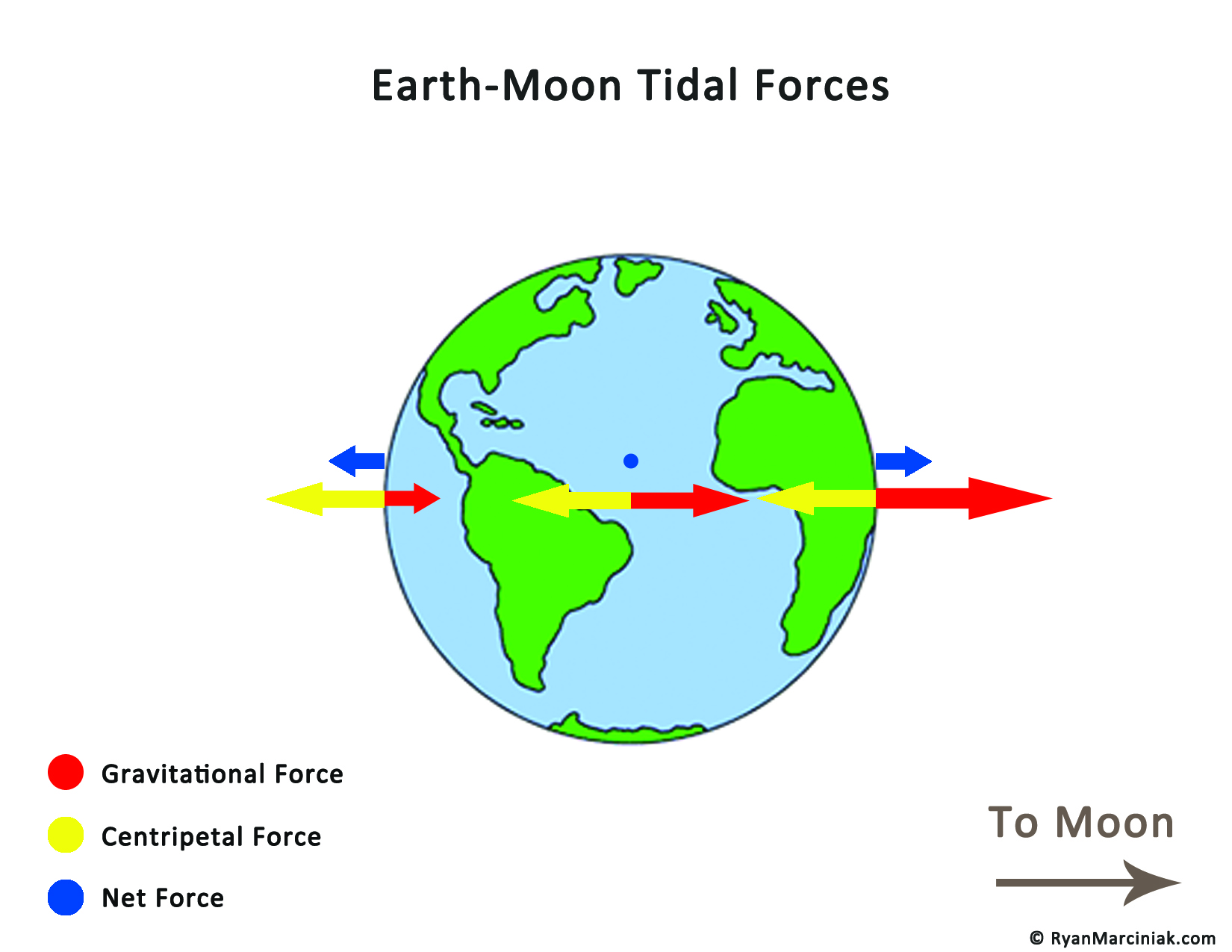
Because the Earth is a large solid body, it’s entire mass orbits at the same speed. This is the key to tidal forces. The Centripetal force of the Earth is the same across it’s entire diameter, but because gravity depends on distance, the side of the Earth facing the Moon feels more gravity that the side facing away. This results in the tidal force on both sides of the Earth, pointing away from the center.
This means that you weigh less when the Moon is above you AND when the Moon is on the opposite side of the Earth from you. In both cases, your weight changes by (almost) the same amount. Here is a map of the tidal force from different points along the Earth’s surface.

So how strong is the tidal force? How much does our weight change because of the Moon?
(Note: If you don’t want to see the math, scroll down to see the answer)
To find the tidal force from the moon, we will compare the Moon’s acceleration due to gravity acting at the edge of the Earth, to that at the center of the Earth. Because we are connected to the Earth, we have the exact same centripetal force, and so we should feel the tidal force acting on us too.
For the side facing the Moon:
Since Force = Mass x Acceleration, we can take Newton’s law of Gravitation from above and divide both sides by mass to get the following formula for acceleration due to gravity.
a = G * M / r2
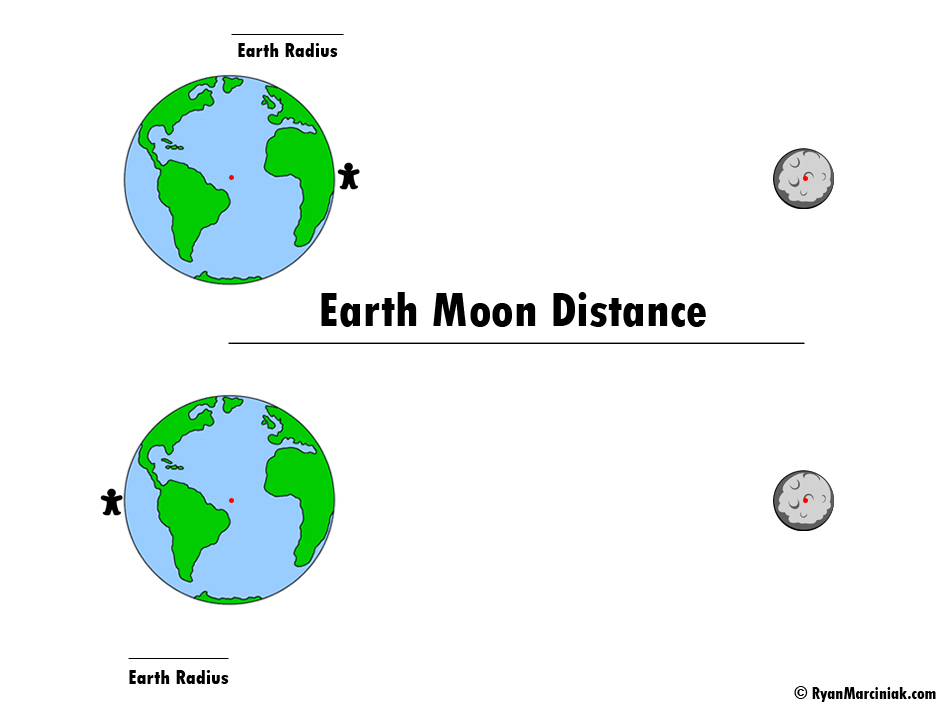
For the edge of the Earth in this case, r will be the Earth-Moon distance (which is measured from the center of the Earth to the center of the Moon) minus the radius of Earth. When we do the calculation for the far side, we will add the Earth’s radius to the Earth-Moon distance.
So if the Earth-Moon distance is R, and Earth’s radius is rEarth,
anear = G * Mmoon / (R – rEarth)2 – G * Mmoon / R2
The Earth-Moon distance is roughly 380,000,000 m, the Earth’s radius is 6,371,000 m, G is G = 6.67 x 10-11N(m/kg)2, and the Moon has a mass of 7.348 x 1022 Kg. The calculation gives us:
anear = 0.00000117 m/s2
A very tiny acceleration.
For the side opposite the Moon:
For the edge of the Earth this time, r will be the Earth-Moon distance plus the radius of Earth. Using the same equation as last time with the new distances, and subtracting the gravity at the edge from that at the center:
afar = G * Mmoon / R2 – G * Mmoon / (R + rEarth)2
With the same numbers as before, the calculation gives us:
afar = 0.00000111 m/s2
A slightly tinier acceleration.
So the tidal force is a little bit stronger on the Moon-facing side, but only by about 5%. Now let’s compare this to the acceleration due to gravity on the Earth from above, 9.81 m/s2.
The gravity you feel from the Moon is just over 1 one-millionth of the gravity you feel from Earth, which means if the Moon is above your head or on the opposite side of Earth, your weight will only drop by a millionth of a Kilogram.

It’s pretty obvious now that the Moon’s gravity won’t give the slightest noticeable effect, and with the Sun and other planets being much further away, their effect is tiny too. The gravity of the Solar System just doesn’t affect us because gravity decreases with the square of the distance. Something that is twice as far from Earth feels only 1/4 of the gravity. So let’s ramp it up a bit. Is there a place in the Solar System where we would notice a difference?
For this one we are going to travel to Jupiter’s Moon Io. Io is the closest of the Galilean moons, and Jupiter is the most massive planet in the solar system. Will we get a different result?
Again the tidal force will make us lighter if Jupiter is overhead or on the opposite side, just as with the Earth and the Moon, but will it make a noticeable difference?
Using Jupiter, the largest planet in the Solar System, and Io, its closest Moon, will Jupiter’s tides make us lighter?

We are going to use the same equation as before, since Newton’s law of gravitation and tidal forces apply to pretty much everything in the Universe (except perhaps the crazy stuff like black holes).
This time the masses are different, as are the distances. We are going to look at how much we weigh on Io, and how much the tidal force is. Start with the general information we will need:
Mass of Io = 8.93 x 1022 Kg
Mass of Jupiter = 1.898 x 1027 Kg
Radius of Io = 1,821,600 m
Jupiter to Io distance (centre to centre) = R = 421,700,000 m
1. Acceleration due to gravity on Io
Let’s forget Jupiter for a second and see what the acceleration due to gravity is on Io:
aIo = G * MIo / (rIo)2
Using the radius and mass of Io from above gives us:
aIo = 1.796 m/s2
Io has a lot less gravity than the Earth, since it’s much smaller. You would weigh around 1/6 of your weight on Earth (similar to the Moon actually).
2. Tidal force due to Jupiter’s gravity
Again we will look at the near and far sides of Io, just as we did when we were standing on Earth. Only this time, it’s mighty Jupiter creating the tides.
For the side with Jupiter above us:
Just as with the Earth and Moon, we will have to find the difference between the gravity at the edge and the gravity at the centre, so we use:
anear = G * MJup / (R – rIo)2 – G * MJup / R2
Where R is the distance between Io and Jupiter. Doing the calculation, we get:
anear = 0.0062 m/s2
For the side opposite Jupiter:
This time, as before, we add the radius of Io to the Jupiter-Io distance to get the correct measure of the tides:
afar = G * MJup / R2 – G * MJup / (R + rIo)2
Where R is the distance between Io and Jupiter. Doing the calculation, we get:
anear = 0.0061 m/s2
A very interesting result!
This means that even with the powerful gravity of Jupiter, your weight on Io will only vary by one ten-thousanth of a Kilogram!
This is because the tidal force really depends on the distance between the objects. As the objects get closer together, the tidal forces increase substantially! If the Moon was twice as close to us as it is now, the tidal forces it exerts on the Earth would increase by a factor of 8!
Even on Io, with Jupiter’s massive gravity, the tides are small because Io is far enough away. If the tides were much stronger, Io would have easily been ripped apart by Jupiter. The minimum distance for a satellite to remain intact is called the roche limit. After this point the tidal force is greater than the satellite’s own gravity, and it is pulled apart.
Io itself is a volcanic world due to tidal forces, but it’s not entirely Jupiter’s fault. The other Galilean Moons of Jupiter contribute to Io having an eccentric orbit, causing Io’s orbital distance to vary. This creates variations in the tidal force it feels from Jupiter, and the changing tides cause Io’s crust to be pushed and pulled regularly, creating friction in the crust and a massive amount of heat that manifests itself as volcanic activity.
It doesn’t get much crazier than this. A special thank you to Jesse Rogerson for double checking my math. Thanks for reading! Don’t ever forget how amazing and crazy and scary the Universe can be!

Very interesting! Thanks
Don’t let Weightwatchers catch wind of this.
really great explanation and article. Keep up the good work
Thank you so much! Feel free to request any topics you would like to see covered!
I think you may have used the wrong word. Centripedal should have been centrifugal. If the force of the person were centripedal it would not be “counteracting” the force of gravity, it would be working with it.
Please let me know if I am correct on this.
Thanx
Harold
Thanks for the comment Harold. You got me thinking about it, and I spent some time digging deeper to try and sort it all out. It turns out (sadly) that we are both wrong.
First off: Centripetal vs. Centrifugal. The analogy I’ll use is a yo-yo swung around your head. As you swing the yo-yo, the important point is that there is a net force acting on it, since it is accelerating by changing direction. This net force is the centripetal force, and it is equal to the tension in the rope. If it was not there, the yo-yo would no longer stay in circular motion, it would fly away in a straight line. The tendency of an object to resist the circular motion is sometimes called the centrifugal force, but it is not a real force. Instead, it is the inertia of the object that causes this straight line tendency. The only real force acting on the yo-yo is the centripetal force from the tension in the rope.
Now in space, we have to distinguish between contact and non-contact forces. Contact forces, sometimes called normal forces, are always acting on us on Earth. Sitting down we feel the chair pushing up on us, resisting gravity. We don’t really feel gravity, we feel the contact force of the ground pushing on us, resisting the force of gravity.
Now here’s where I was wrong. I associated free fall and weightlessness with zero net force. An astronaut in orbit is accelerating, they have to be, the direction of their motion is constantly changing as they move in an elliptical orbit around the Earth. So they have a net force, which is the centripetal force, and is also the gravitational force, they are one and the same in this case, as with tension in the yo-yo analogy. But there are no contact forces, nothing pushing on them that allows them to feel the force of gravity. That’s what free fall is. It’s not the absence of gravity, it’s the absence of contact forces. It’s Einstein’s relativity in action.
One more point to consider to explain this. If I were in an elevator that was falling toward the Earth, I can’t see anything outside of the elevator, so I feel weightless, and I could float around in the elevator. Gravity is still acting upon myself and the elevator, but there are no contact forces from the ground or anything.
I hope this makes sense, and I thank you for your comment! I’ll make the changes to the post to clear up the confusion in my original explanation.
I guess you could think of the astronauts as being in “free fall” but I think it is more like a state of equilibrium.
“free Fall” would be more accurate to describe an object that is traveling at 0 miles per hour in comparison to the earth’s surface (tangent to the pull of gravity), therefore it would instantly accelerate toward the earth “freely” with no Centrifugal forces fighting gravity.
The astronauts, on the other hand are traveling at a high rate of speed tangent to the earths gravitation. If this speed is increased they will “Escape” the earths gravity and shoot off into space… if this speed is decreased they would descend to the earths atmosphere where they would slow quickly due to friction of atmosphere and burn to the earths surface.
Summary: astronauts are not in free fall – they may be falling – but the major force that is keeping them stable is the energy that accelerated them to their orbital speed combined with the lack of anything to slow them down.
Thanx for your post and site and everything you do to educate people! ! !
Harold
Thanks for the comment Harold – I think this one will be solved by my answer to your other comment. Happy to discuss it a bit further if you like.
I have a Question,
When in space you can create an artificial gravity by having the entity that you are in, spin. The force is on you pushing toward your feet, as you are walking with your head facing the center of the centrifuge. But how about the moon revolving around the earth and not spinning. Wouldn’t the gravity be different on one side of the moon than the side facing the earth?
Great question!
First off the Moon actually is spinning! But it’s what we call ‘tidally locked’ to Earth, so it spins in the same amount of time it takes to orbit, 29.5 days. To picture this, imagine that it’s the Earth orbiting the Moon instead of the other way around. If we were to always see the same face of the Moon, it would have to spin as we orbited around it.
“Wouldn’t the gravity be different on one side of the moon than the side facing the earth?”
It is, also because of the tidal force, except this time it’s Earth exerting it on the Moon, but again these tidal forces are too small to make a difference to a tiny human. As for the centripetal forces, the difference in the angular speed from the near to the far sides of the Moon are tiny, and so the resulting difference in centripetal forces are tinier than even the tidal force I discussed in the article.
Let me know if I understood your questions correctly – happy to answer if you have follow up.
Thanks so much for this… I Googled exactly this, as I wondered if I was lighter when the moon was overhead.
Your explanation was very thorough and then you preempted a further question when you did all the math again on Io 😀
I wish you had been my science teacher!
That’s really nice of you to say – thank you!
Would you be weightless at the center of the earth as mass is equal in all directions?
Great question! You would be weightless at the Earth’s center of gravity, which may not be the geological center, as the density of Earth does vary, meaning the gravitational field does too. It’s not a huge variation, but enough that we can measure it with scientific instruments.
Hi there, just a quick question regarding your conclusion
“The gravity you feel from the Moon is just over 1 one-millionth of the gravity you feel from Earth, which means if the Moon is above your head or on the opposite side of Earth, your weight will only drop by a millionth of a Kilogram.”
If your weight = your mass * acceleration due to gravity, surely that statement should read “your weight will only drop by a millionth of your mass”?
Hmmm you are correct, but I find your statement makes it more confusing. What is changing is the net force on your body, which is what we measure as weight. You correctly pointed this out. But it makes it easier to understand if you compare a person’s weight with and without the Moon’s effect. The layperson can envision this when thinking about measuring their weight on a scale, in Kilograms.
It is not one-millionth, Your weight on the Moon is 16.5% what you would experience on Earth. In other words, if you weighed 100 kg on Earth, you would weigh a mere 16.5 kg on the Moon. For you imperial folks, imagine you tipped the scales at 200 pounds. Your weight on the Moon would only be 33 pounds.
Hi Jesse,
You are correct if you are on the surface of the Moon, where the surface gravity is 1/6th of the Earth’s surface gravity. However, we are talking about the effect of the Moon’s gravity when you are on the surface of the Earth. This is where the Moon’s gravitational effect on a person is one-millionth that of the Earth.
Thanks for the comment!
Hi,
I think that your article is really well written. Thank you for the effort!
I think the point Sam made is actually important: The weight difference you would see on the scale depends on your mass.
For example, if your weight is 50 kg, the difference would be 50/1million kg. If you want o make the statement easier to understand for a layperson, you can give an example like that. To suggest that the difference would be the same for everybody could lead to serious misunderstandings.
It’s just a detail, apart from that I very much enjoyed reading the article!!
Greetings
Thanks for the feedback!
I’m keeping a link to this site in my Tiddlywiki for future use. Every so often I see a question or comment on Quora, Youtube comments, or some physics or sci fi forum on tidal forces, how much lighter you are on a bathroom scale at noon during a new moon vs at sunrise, and so on. This page does a fine job with illustrations, explanations and working the numbers.
Thanks for the comment! Glad my writing is useful to you.